Heavy vector and axial-vector D mesons in hot magnetized asymmetric nuclear matter

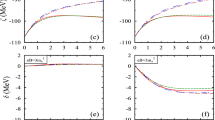
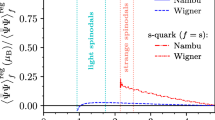
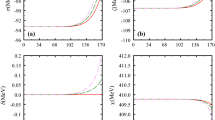
Abstract

We observed the impact of the finite magnetic field on the in-medium mass and decay constant of isospin averaged vector $D^*(D^{*^+},D^{*^0})$ and axial-vector $D_1(D^+_1, D^0_1)$ mesons. The quark and gluon condensates of the nuclear medium at the finite magnetic field, temperature, isospin asymmetry, and density have been obtained by the meson exchange scalar fields within the chiral SU(3) model. The medium attributes modify the scalar and vector density of the nuclear medium and this variation reflects in the in-medium mass and decay constant of spin 1 D mesons. We calculate these observables by comparing the Operator Product Expansion (OPE) and the phenomenological side in the QCD Sum Rules. In the results, we observed a positive mass shift for charged vector and axial-vector D mesons concerning the magnetic field. For neutral vector (axial-vector) D mesons we observed negative (positive) mass shift as a function of the magnetic field. In the application part, we calculate the in-medium partial decay width of the process $D^*_s$(2715/2860) $\rightarrow$ $D^* K$ by using $^3P_0$ model. The in-medium effects are incorporated through the in-medium masses of $D^*$ and K mesons.

Appendix A: Even-odd QCD Sum Rules

QCD Sum Rules are another formalism to study the physics of non-perturbative regime [32,33,34]. This formalism is based on the Borel transformation which is a mathematical technique to avoid the divergence in the perturbative expansion [34, 36]. To relate the mesons properties from medium to the vacuum, we start with the two-point current co-relation function

$$\begin{aligned} \Pi (p) = i \int d^4y \, e^{ipy} \left\langle \langle {\hat{O}} |\text {T} \left[ \text {j} \right] (y) \text {j}^\dagger (0) | {\hat{O}} \rangle \right\rangle \end{aligned}$$

(A1)

which represents time-ordered product of the meson currents $\text {j}(y)$ and $\text {j}^\dagger (0)$. To study the in-medium mass of $D^+$ and $D^-$ mesons separately, we split the co-relation function $\Pi (p_0,\mathbf {p}\,)$ into an even (e) and odd (o) part as

$$\begin{aligned} \Pi (p_0,\mathbf {p}\,) = \Pi _e(p^2_0,\mathbf {p}\,) + p_0 \Pi _o(p^2_0,\mathbf {p}\,). \end{aligned}$$

(A2)

Explicitly,

$$\begin{aligned} \Pi _e(p_0,\mathbf {p}\,)&= \frac{1}{2} \left( \Pi (p_0,\mathbf {p}\,) + \Pi (-p_0,\mathbf {p}\,)\right) = \Pi _e(-p_0,\mathbf {p}\,) \, , \end{aligned}$$

(A3)

and

$$\begin{aligned} \Pi _o(p_0,\mathbf {p}\,)&= \frac{1}{2p_0} \left( \Pi (p_0,\mathbf {p}\,) - \Pi (-p_0,\mathbf {p}\,)\right) \nonumber \\&= \Pi _o(-p_0,\mathbf {p}\,) \, . \end{aligned}$$

(A4)

In the rest frame, the OPE side is derived using pseudoscalar current $\text {j}_{D^+} = i {\overline{d}} \gamma _5 c$ and $\text {j}_{D^-} = \text {j}^\dagger _{D^+}(x) = i {\overline{c}} \gamma _5 d$. By application of the Borel transformation operator on the OPE side with the condition of large pole mass of charm quark $m_c$ and $m_d \rightarrow 0$, we obtain

$$\begin{aligned}&{\mathcal {B}} \left[ \Pi _e^{OPE}(\omega ^2, \mathbf {p} = 0\,) \right] \left( M^2 \right) \nonumber \\&= \frac{1}{\pi } \int _{m_c^2}^\infty ds e^{-s/M^2} \text {Im} \Pi ^{per}(s, \mathbf {p} = 0\,) + e^{-m_c^2/M^2} \nonumber \\&\qquad \left( -m_c \langle {\overline{d}}d \rangle + \frac{1}{2} \left( \frac{m_c^3}{2M^4} - \frac{m_c}{M^2} \right) \langle {\overline{d}}g\sigma {\mathcal {G}}d \rangle \right. \nonumber \\&\quad + \frac{1}{12} \langle \frac{\alpha _s}{\pi } G^2 \rangle + \left[ \left( \frac{7}{18} + \frac{1}{3} \ln \frac{\mu ^2 m_c^2}{M^4} - \frac{2\gamma }{3} \right) \right. \nonumber \\&\qquad \left. \left( \frac{m_c^2}{M^2} - 1 \right) - \frac{2}{3} \frac{m_c^2}{M^2} \right] \langle \frac{\alpha _s}{\pi } \left( \frac{(vG)^2}{v^2} - \frac{G^2}{4} \right) \rangle \nonumber \\&\quad + 2 \left( \frac{m_c^2}{M^2} - 1 \right) \langle d^\dagger iD_0 d \rangle + 4 \left( \frac{m_c^3}{2M^4} - \frac{m_c}{M^2} \right) \nonumber \\&\quad \left. \left[ \langle {\overline{d}} D_0^2 d \rangle - \frac{1}{8} \langle {\overline{d}} g \sigma {\mathcal {G}} d \rangle \right] \right) \, , \end{aligned}$$

(A5a)

$$\begin{aligned}&{\mathcal {B}} \left[ \Pi _o^{OPE}(\omega ^2, \mathbf {p} = 0\,) \right] \left( M^2 \right) \nonumber \\&\quad = e^{-m_c^2/M^2} \left( \langle d^\dagger d \rangle - 4 \left( \frac{m_c^2}{2M^4} - \frac{1}{M^2} \right) \right. \nonumber \\&\quad \left. \langle d^\dagger D_0^2 d \rangle - \frac{1}{M^2} \langle d^\dagger g \sigma {\mathcal {G}} d \rangle \right) , \end{aligned}$$

(A5b)

where

$$\begin{aligned}&{\text {Im}} \Pi ^{p e r}\left( p^{2}\right) \nonumber \\&\quad = \frac{3}{8 \pi } \frac{\left( p^{2}-m_{c}^{2}\right) ^{2}}{p^{2}}\left\{ 1+\frac{4 \alpha _{s}\left( p^{2}\right) }{3 \pi }\left[ \frac{9}{4}+2 {\text {Li}}_{2}\left( \frac{m_{c}^{2}}{p^{2}}\right) \right. \right. \nonumber \\&\qquad + \ln \left( \frac{p^{2}}{m_{c}^{2}}\right) \ln \left( \frac{p^{2}}{p^{2}-m_{c}^{2}}\right) \nonumber \\&\qquad +\frac{3}{2} \ln \left( \frac{m_{c}^{2}}{p^{2}-m_{c}^{2}}\right) +\ln \left( \frac{p^{2}}{p^{2}-m_{c}^{2}}\right) \nonumber \\&\qquad \left. +\frac{m_{c}^{2}}{p^{2}} \ln \left( \frac{p^{2}-m_{c}^{2}}{m_{c}^{2}}\right) +\frac{m_{c}^{2}}{p^{2}-m_{c}^{2}} \ln \left( \frac{p^{2}}{m_{c}^{2}}\right) \right] . \end{aligned}$$

(A6)

In the above equation, ${\text {Li}}_{2}(y)=-\int _{0}^{y} z^{-1} \ln (1-z) d z$ is the Spence function.

The physical quantity mentioned inside $\langle \rangle _{\rho _N}$ in Eqs. (A5b) and (A5a) are known as condensates. The density dependent down quark condensate, $\langle {\overline{d}}d \rangle _{\rho _N}$ and scalar gluon condensate, $\langle \frac{\alpha _s}{\pi } G^2 \rangle _{\rho _N}$ are calculated from chiral SU(3) model by Eqs. (23) and (27), respectively and the values of other in-medium quark and gluon condensates are taken from Ref. [33]. The numerical values of these in-medium condensates along with other sum rules parameters are mentioned in the Table 6. In the Borel transformed sum rules of pseudoscalar D meson, the charm quark mass, $m_c$ plays a crucial role to intensify the contribution of light quark condensate, $\langle {\overline{d}}d \rangle _{\rho _N}$.

The in-medium sum rules for the even part up to mass dimension 5, can be rewritten in the functional form [33, 98]

$$\begin{aligned}&h\left( M, s_{0}, m_{c}, \mu \right) \equiv m_+ H_{+} e^{-m_{+}^{2} / M^{2}}+m_{-}H_{-} e^{-m_{-}^{2} / M^{2}} \nonumber \\&\quad =\frac{1}{\pi } \int _{m_{c}^{2}}^{s_{0}} d s e^{-s / M^{2}} {\text {Im}} \Pi ^{p e r}(s) \nonumber \\&\qquad +e^{-m_{c}^{2} / M^{2}}\left( -m_{c}\langle {\bar{d}} d\rangle _{\rho _N}+\frac{1}{2}\left( \frac{m_{c}^{3}}{2 M^{4}}-\frac{m_{c}}{M^{2}}\right) \right. \nonumber \\&\qquad \langle {\bar{d}} g \sigma {\mathcal {G}} d\rangle _{\rho _N}+\frac{1}{12}\left\langle \frac{\alpha _{s}}{\pi } G^{2}\right\rangle _{\rho _N} \nonumber \\&\qquad +\left[ \left( \frac{7}{18}+\frac{1}{3} \ln \frac{\mu ^{2} m_{c}^{2}}{M^{4}}-\frac{2 \gamma }{3}\right) \left( \frac{m_{c}^{2}}{M^{2}}-1\right) -\frac{2}{3} \frac{m_{c}^{2}}{M^{2}}\right] \nonumber \\&\qquad \left\langle \frac{\alpha _{s}}{\pi }\left( \frac{(v G)^{2}}{v^{2}}-\frac{G^{2}}{4}\right) \right\rangle _{\rho _N} \nonumber \\&\qquad +2\left( \frac{m_{c}^{2}}{M^{2}}-1\right) \left\langle d^{\dagger } i D_{0} d\right\rangle _{\rho _N}+4\left( \frac{m_{c}^{3}}{2 M^{4}}-\frac{m_{c}}{M^{2}}\right) \nonumber \\&\qquad \left[ \left\langle {\bar{d}} D_{0}^{2} d\right\rangle _{\rho _N}-\frac{1}{8}\langle {\bar{d}} g \sigma {\mathcal {G}} d\rangle _{\rho _N}\right] , \end{aligned}$$

(A7)

where $s_0$ is the continuum threshold parameter and its density dependence is given by the relation, ${s^*_0}^2=s^2_0 \pm \frac{\rho _N}{\rho _0}$ [33].

Now, the in-medium sum rules for the odd part can be written as

$$\begin{aligned} s\left( M, m_{c}\right)&\equiv H_{+} e^{-m_{+}^{2} / M^{2}}-H_{-} e^{-m_{-}^{2} / M^{2}}\nonumber \\&=e^{-m_{c}^{2} / M^{2}}\left( \left\langle d^{\dagger } d\right\rangle _{\rho _N}-4\left( \frac{m_{c}^{2}}{2 M^{4}}-\frac{1}{M^{2}}\right) \right. \nonumber \\&\quad \left. \left\langle d^{\dagger } D_{0}^{2} d\right\rangle _{\rho _N}-\frac{1}{M^{2}}\left\langle d^{\dagger } g \sigma {\mathcal {G}} d\right\rangle _{\rho _N}\right) . \end{aligned}$$

(A8)

Using the above two equations, the in-medium mass of $D^+$ and $D^-$ can be evaluated using the following formula [98]

$$\begin{aligned} m^*_{\pm }&=\left[ \frac{1}{4}\left( \frac{s\left( M, m_{c}\right) \frac{d}{dz} h\left( M, s_{0}, m_{c}, \mu \right) -h\left( M, s_{0}, m_{c}, \mu \right) \frac{d}{dz} s\left( M, m_{c}\right) }{h^{2}\left( M, s_{0}, m_{c}, \mu \right) +s\left( M, m_{c}\right) \frac{d}{dz} s\left( M, m_{c}\right) }\right) ^{2}\right. \nonumber \\&\quad \left. -\frac{h\left( M, s_{0}, m_{c}, \mu \right) \frac{d}{dz} h\left( M, s_{0}, m_{c}, \mu \right) +\left( \frac{d}{dz} s\left( M, m_{c}\right) \right) ^{2}}{h^{2}\left( M, s_{0}, m_{c}, \mu \right) +s\left( M, m_{c}\right) \frac{d}{dz} s\left( M, m_{c}\right) }\right] ^{\frac{1}{2}}\nonumber \\&\quad \pm \frac{1}{2} \frac{s\left( M, m_{c}\right) \frac{d}{dz} h\left( M, s_{0}, m_{c}, \mu \right) -h\left( M, s_{0}, m_{c}, \mu \right) \frac{d}{dz} g\left( M, m_{c}\right) }{h^{2}\left( M, s_{0}, m_{c}, \mu \right) +s\left( M, m_{c}\right) \frac{d}{dz} s\left( M, m_{c}\right) }, \end{aligned}$$

(A9)

where $z=1 / M^{2}$, with M as a Borel mass parameter. Clearly, the in-medium mass is a function of M and to get a physical valid mass one should choose a proper Borel Window [98]. To reproduce the properties of hadronic medium, there should be balanced contribution from “pole + continuum” ansatz i.e the perturbative contributions from the continuum part should not influence the result in a larger extent. The higher excitations are controlled by the exponential factor in the perturbative integral which make sure that the continuum contribution to the spectral integral is less than the pole contribution. The expression to calculate maximum Borel mass, $M_{max}$ is given as

$$\begin{aligned} 1 \ge \frac{\frac{1}{\pi } \int _{s_{0}}^{\infty } d s {\text {Im}} \Pi ^{p e r}(s) e^{-s / M_{\max }^{2}}}{h\left( M_{\max }, s_{0}, m_{c}, \mu \right) }. \end{aligned}$$

(A10)

For the lower bound $M_{min}$ of the Borel window, the highest mass dimension terms, should not provide contribution greater than 10$\%$ to the OPE side of sum rule. This condition is implied by the following inequality

$$\begin{aligned} 0.1 \ge \frac{e^{-m_{c}^{2} / M_{\min }^{2}}\left( \frac{m_{c}^{3}}{4 M_{\min }^{4}}\langle {\bar{d}} g \sigma {\mathcal {G}} d\rangle +2 \frac{m_{c}^{3}}{M_{min}^{4}}\left[ \left\langle {\bar{d}} D_{0}^{2} d\right\rangle -\frac{1}{8}\langle {\bar{d}} g \sigma {\mathcal {G}} d\rangle \right] \right) }{\frac{1}{\pi } \int _{s_{0}}^{\infty } ds \text {Im} \Pi ^{pe r}(s) e^{-s /M_{min}^{2}}+h \left( M_{min}, s_0, m_{c}, \mu \right) }.\nonumber \\ \end{aligned}$$

(A11)

Further, the mass-splitting between charged partners ($D^+, D^-$) can be calculated as

$$\begin{aligned} \Delta m^*=m^*_+-m^*_- \end{aligned}$$

(A12)

Table 6 List of used parameters